So here's the answers to week 1's problems.
1. 48 (ARML/2010)
2. 1006 (SMO(S)/2011)
Solution (1):
Assuming you know the formula for circles and straight lines (if you don't look it up), we have the following diagram.
Points C,F,E,D lie on the circle, and A,D are the midpoints of CE,DF respectively. B is the intersection of CE,DF .
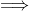AB=4 and BG=3
It seems impossible to determine the areas individually because of the lack of information.
Since we are asked to compute
, we see that some parts of the circle cancels out each other.
Inspired by this, we construct lines x=-4 and y=-3.
Then, we have
Since
, 
, 
, we have
Solution (2):
It should be obvious that we need to solve this series by pairing terms. We notice that the series can be paired as
Because each pair of terms is of the form
1. 48 (ARML/2010)
2. 1006 (SMO(S)/2011)
Solution (1):
Assuming you know the formula for circles and straight lines (if you don't look it up), we have the following diagram.
Points C,F,E,D lie on the circle, and A,D are the midpoints of CE,DF respectively. B is the intersection of CE,DF .
It seems impossible to determine the areas individually because of the lack of information.
Since we are asked to compute
Inspired by this, we construct lines x=-4 and y=-3.
Then, we have
Since
Solution (2):
It should be obvious that we need to solve this series by pairing terms. We notice that the series can be paired as
Because each pair of terms is of the form
, which is equal to exactly 1.
There are 1006 pairs of terms, thus the sum is 1006.
